Author
Math Country
Share
Author
Math Country
Share
One lesson – several topics
Today we would like to share our experience in teaching elementary math and how to utilize one of the best math teaching strategies in your everyday practice. As a team of practicing tutors, we are always looking for practice and strategies to improve the results of our work. Our favorite and one of the most effective math teaching strategies is topic rotation, or covering several topics in one lesson.
Cover several topics in one lesson
Why and how covering several topics in one lesson is beneficial for children rather than the typical “one lesson = one topic” method?
We want to point out that we are not talking about absolutely new concepts to children. We are talking about deepening and consolidating existing skills. Entirely new concepts require a whole lesson of practice and explanations.
Our brains are designed in such a way that we forget things we don’t use. Children learn much faster and easier than adults. Unfortunately, they also forget more quickly. If children do not use the skill for several weeks, the skill vanishes from their minds.
Humans have several types of memory, including short-term and long-term memory. As educators, we aim to ensure that the necessary skill moves from short-term memory into children’s long-term memory. This process happens only during sleep. It is scientifically proven that we need good sleep to learn.
After a new skill moves from short-term memory into long-term memory, we must refer to it to ensure that it stays useful and that we remember it. The more times we refer to a skill, the stronger brain connections are built, and the more likely our memory will save it instead of overriding it with new information.
This is why the practices “finish one topic before starting another” and “one topic = one lesson” do not help the child.
Let’s see what happens if we use this common math teaching strategy.
Common mistakes and problems
Problem # 1:
Children start to learn fractions. They learn what it is, learn to split shapes into parts, add/subtract fractions, show them on a number line, etc. Children learn something new daily for two weeks, repeat what they learned yesterday, and refer to the new skills. They take a test at the end and do great.
After this, children start to learn decimals, and everything repeats. They take a decimals test and do great again.
The problem is that children forget almost everything about fractions. If children take a fractions test at this point, their results will decrease drastically. To bring to life their previous fractions knowledge, they would have to spend about ½ of the time they spent when they started learning fractions.
Problem #2:
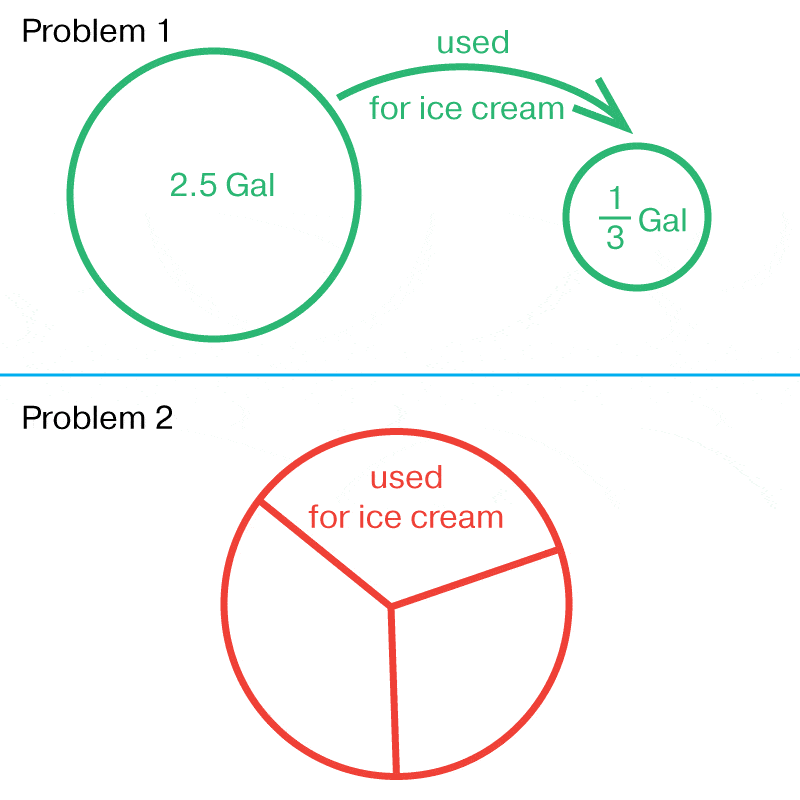
Children are used to the practice of “one lesson = one topic”. Let’s suppose the topic is “Multiplying Fractions”. Children repeat and learn the rules and practice them with some simple problems. Now it is time to solve word problems. You may not notice anything if you continue with the “one lesson = one topic” practice. But if you put several problems on addition/subtraction of fractions, you will see that most children make mistakes when solving these problems. They did not read the whole problem, instead they picked up numbers and multiplied them.
What does this tell us?
Children have already made a pattern for the lesson and followed it. They do not use (or refer to) previously learned skills; they do not learn anything new because following the pattern does not require using knowledge. Plus, children will forget this pattern as soon as the lesson ends because they will need a new pattern for the next lesson, so there is no need to memorize this one. Then what was the point of the lesson?
As you see, topic rotation is not only beneficial but essential for productive lessons.
We also need to mention, that this math teaching strategy effective not only for neurotypical children, but also useful for children with AD(H)D disorder.
We made a short check-list to help you adopt and apply this math teaching practice.
How to teach elementary math effectively:
- Keep children focused with topics rotation;
- Alternate complex exercises with simple ones;
- Include word and logic problems;
- Ask children to explain the solution.
Stay in the loop