Fraction of a number. How to explain it

Fractions are one of the most difficult topics in elementary school. It gets even more difficult if students do not completely understand fractions and what to do with them. While it is not hard to learn the rules on how to add, subtract, multiply, and divide fractions, it is quite difficult to solve fraction multiplication word problems.

How to find a fraction of a number

Most students can find a fraction of a number if it is a singular worksheet problem. However, it is common for most students to struggle to solve word problems when they must find a part of a number or a fraction.

Most US school curricula start with teaching to multiply a fraction by another fraction. Children must memorize and follow the given rule (numerator × numerator over denominator × denominator).

After that, children learn to multiply a fraction by a whole number by converting a whole number into a fraction with the denominator of 1 and then using the same rule.

It does look easy to memorize and follow the rule, but it does not give children any idea or understanding of why and how it works; they blindly apply the rule without understanding the context.

We would not deny that most children would prefer to memorize and use the rule instead of understanding how it works because this is one of the most challenging issues in elementary school mathematics. So, why do we need all these fraction of a number worksheet explanations if it is easier for teachers and children to memorize a simple rule?

We do not want to teach children to use some not understandable or random rules one after another to get the desired result. If the rule is unclear, nobody can predict a result. Our priority is not making children calculate correctly (people constantly use calculators for this reason). We want them to learn to find a correct solution for a problem, which is only possible with understanding. If you want children to master fraction multiplication word problems, you have to follow several steps.

The following steps help children understand, adopt, and use this concept.

1. The best way to start is to multiply a fraction by a whole number. Do not give any rules to the child. Ask them to use addition to get an answer. After several problems, children clearly see and understand the pattern and are ready to voice the rule themselves.
2. Stop at this point and focus children’s attention on what happens with a fraction if we multiply it by a whole number: the result is greater than the beginning fraction (we are talking about positive proper fractions).
3. Now, since multiplication is a commutative operation (it means that 5×9=9×5), switch places of a fraction and a whole number. For example, if you have 1/6 × 5 = 5/6, ask children to find the result of 5 × 1/6. They already know it. Focus their attention on what happens with a whole number if we multiply it by a (proper) fraction: the result is less than the beginning whole number.

These are two main concepts children should understand (applies to positive numbers only) while practicing multiplying fractions and whole numbers:

• When we multiply a fraction (or a number) by a number greater than one, the result is greater than the starting number.
• When we multiply a number (or a fraction) by a number less than one, the result is less than the starting number.

The next step is to use a pizza model.

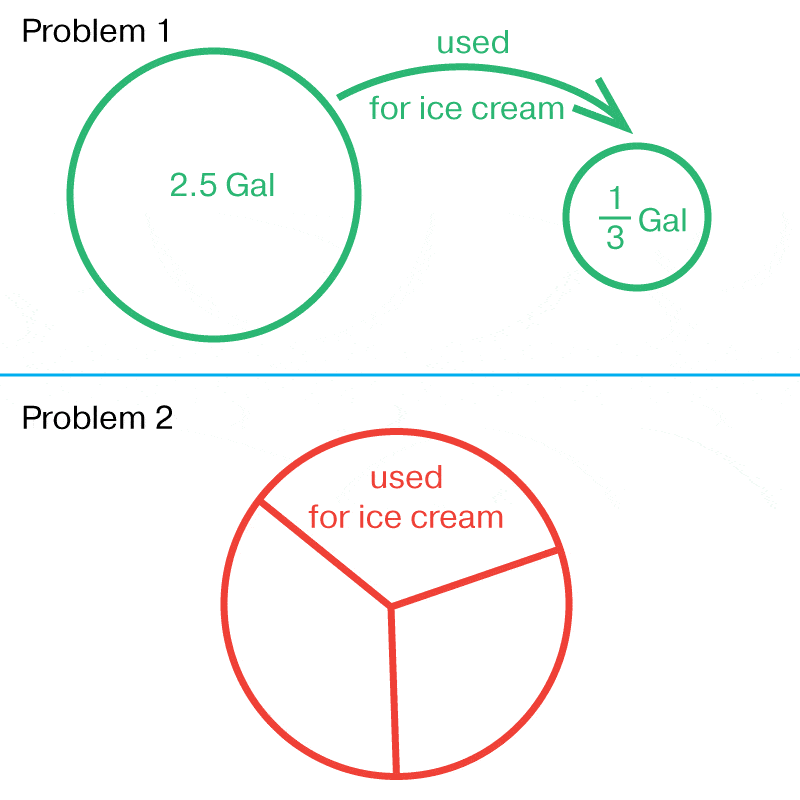
1. Draw a circle and ask children to find 1/3 of the model. They already know they must split a model into three equal parts and take one.
2. Draw the same circle and ask children to find 1/3 of these two models. Most likely, children split the second model into three parts and take one of the pieces from each model, which is equal to 2/3. Write the result.
3. Continue to add the pizza model and ask to find 1/3 of the whole amount. Very soon, children get the pattern and give you the correct answer but are not ready yet to make the rule.
4. Finally, instead of drawing 7 pizza models, you can draw one circle and tell children it represents 7 pizzas. Ask them to find 1/5 of this. By this time, children easily give the correct answer.

Do not forget to focus their attention on how the result differs from the starting numbers of pizza.

Write all of that thinking process as expressions:

• To find 2/3 of a pizza, we must split a pizza into 3 equal parts (this is a division) and take two parts (multiplication): 1 ÷ 3 × 2.
• To find 2/3 of 5 pizzas. we have to take 2/3 of each of these pizzas and multiply by 5: 2/3 × 5. Since multiplication is commutative, we can rewrite it as 5 × 2/3.
• Repeat the whole chain as many times as children need to understand this replacement and can repeat it without your help.

When children understand this concept entirely, it is time to practice it with fraction multiplication word problems.

To help children fully understand and master Fractions topic, we specially designed our fraction worksheets grades 2-4. Parents and teachers can use these worksheets for practice fractions problems in class or at home.

Grade 2 Fractions Worksheets

Grades 3-4 Fractions worksheets